Rotational kinetic Energy of Disc and Hoop

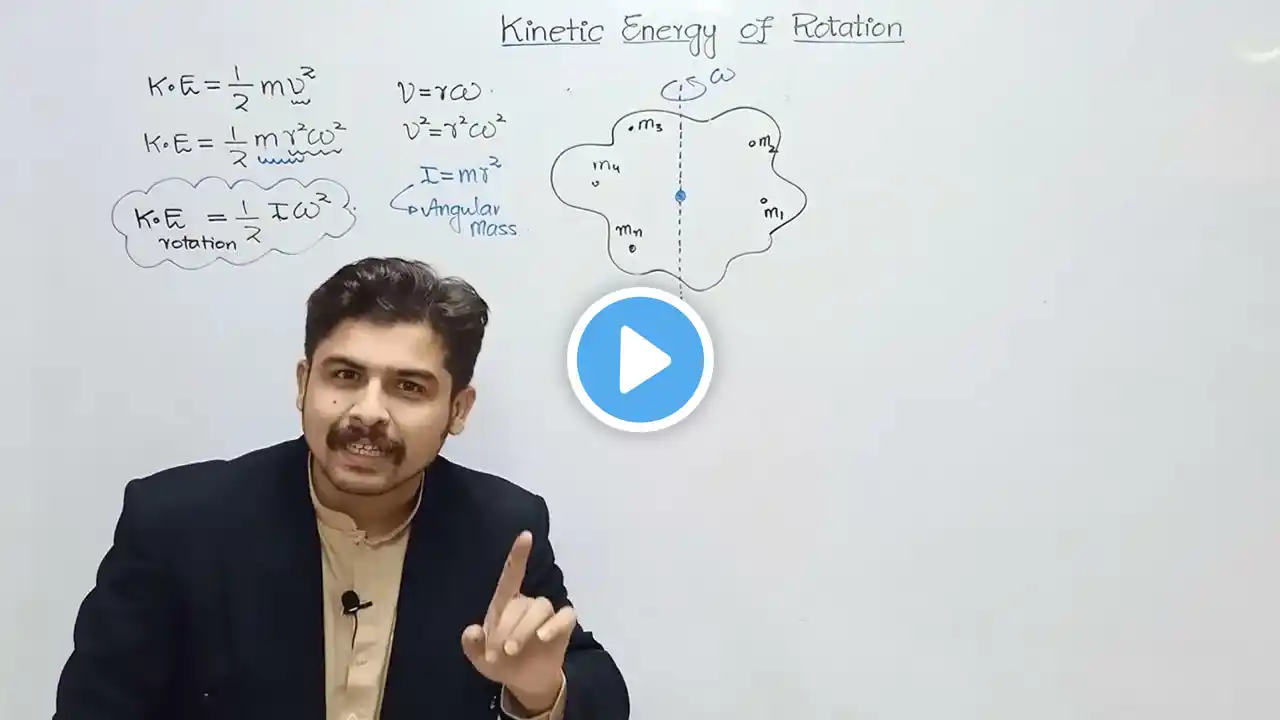
Rotational kinetic energy is the energy an object possesses due to its rotation around an axis. For both a disc and a hoop, this energy can be described using the formula: \[ KE_{rot} = \frac{1}{2} I \omega^2 \] where: \( KE_{rot} \) is the rotational kinetic energy, \( I \) is the moment of inertia, and \( \omega \) is the angular velocity. Disc **Moment of Inertia**: For a solid disc of mass \( m \) and radius \( r \), the moment of inertia is given by: \[ I_{disc} = \frac{1}{2} m r^2 \] **Rotational Kinetic Energy**: Therefore, the rotational kinetic energy of a disc can be expressed as: \[ KE_{rot, disc} = \frac{1}{2} \left( \frac{1}{2} m r^2 \right) \omega^2 = \frac{1}{4} m r^2 \omega^2 \] Hoop **Moment of Inertia**: For a hoop (or thin ring) of mass \( m \) and radius \( r \), the moment of inertia is: \[ I_{hoop} = m r^2 \] **Rotational Kinetic Energy**: Thus, the rotational kinetic energy of a hoop is: \[ KE_{rot, hoop} = \frac{1}{2} (m r^2) \omega^2 = \frac{1}{2} m r^2 \omega^2 \] Comparison **Moment of Inertia**: The hoop has a larger moment of inertia compared to the disc because all of its mass is distributed at the radius \( r \), whereas the disc has its mass distributed throughout its volume. **Kinetic Energy**: This difference in moment of inertia leads to the hoop having greater rotational kinetic energy for the same mass and angular velocity. Understanding these differences is essential in applications involving rotational dynamics, such as in engineering and physics problems involving rotating bodies.